A mere acquaintance with double-entry book-keeping indicates, indeed, that the only place where people can put their money is into someone else’s pocket. When Mr. X buys shares from Mrs. Y for cash, the former substitutes shares for cash in his assets, while the latter does the opposite in her assets. Money has not disappeared, it has just changed hands. In an extreme case, if market participants were to hoard the whole of their money balances, by design not a single transaction would take place and there would not be any asset price to observe. Therefore, it is not the quantity of money but its circulation that causes asset prices to rise or to fall. Yet, few people seem to consider the velocity of money in capital markets as a variable of interest.
Measurement issues are at least partly responsible for this neglect.
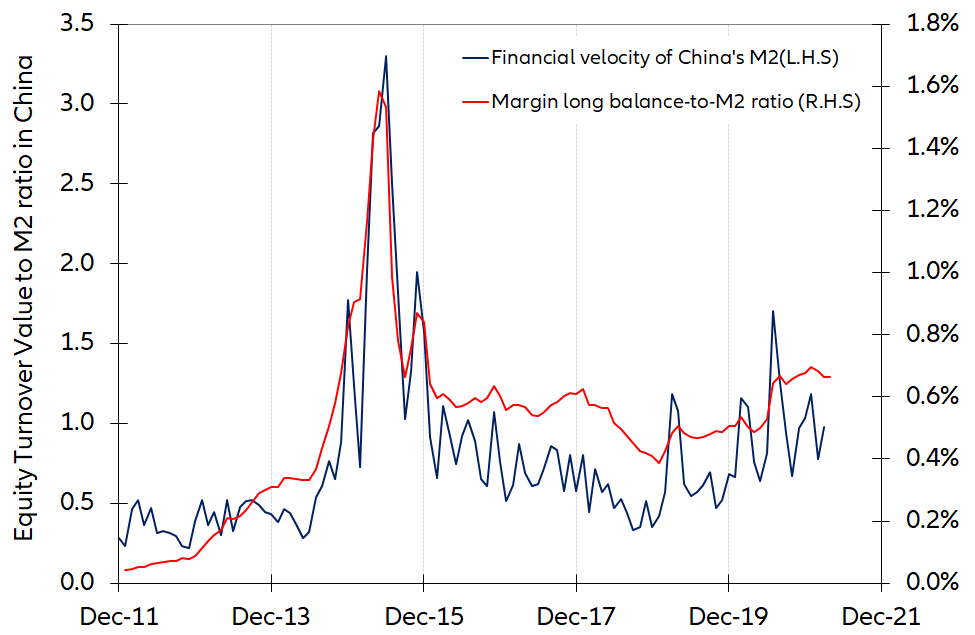
One cannot comprehensively observe how frequently money changes hands in all segments of capital markets. The nominal value of transactions executed in stock exchanges is usually known, but that is not the case for transactions executed in OTC markets or dark pools. As for the share of the money supply involved in financial transactions, it is anybody’s guess. For lack of a more comprehensive and granular measure, we shall define the financial velocity of money as the ratio of the equity turnover value to the broad money supply.
The unbearable fickleness of financial velocity. The neglect of the financial velocity of money is all the more puzzling as there is some evidence that central banks do not seem to control it. China is a case in point. Why investigate this issue in China? Because the observation conditions are probably better in China than elsewhere. Chinese equity markets and money supply have been and still are less open to foreign influence than their US counterparts. Chinese data on the nominal value of transactions are probably more comprehensive and reliable than the US ones.
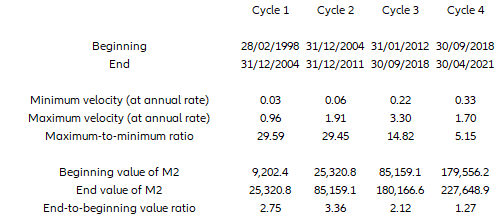
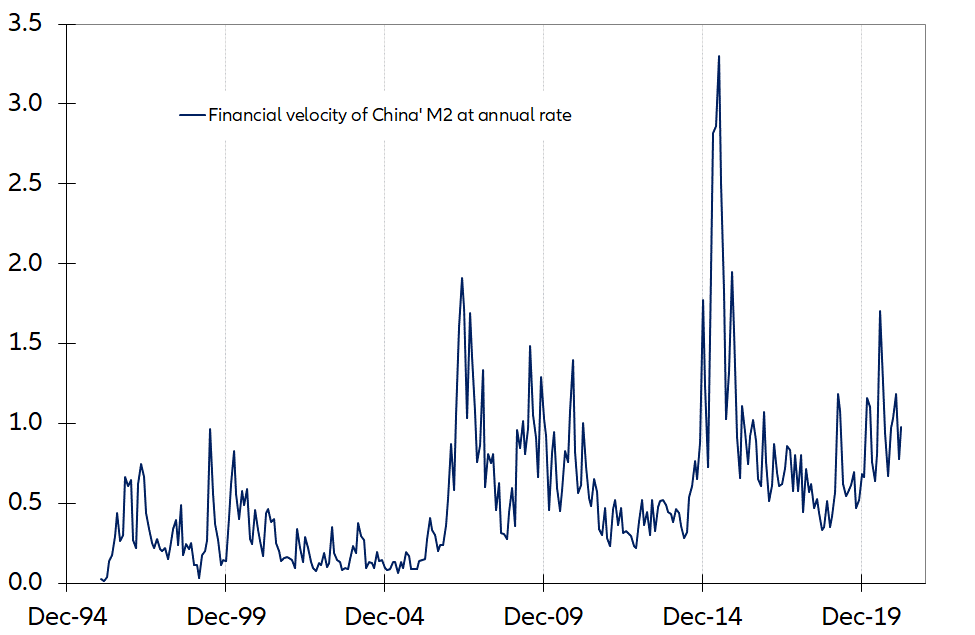
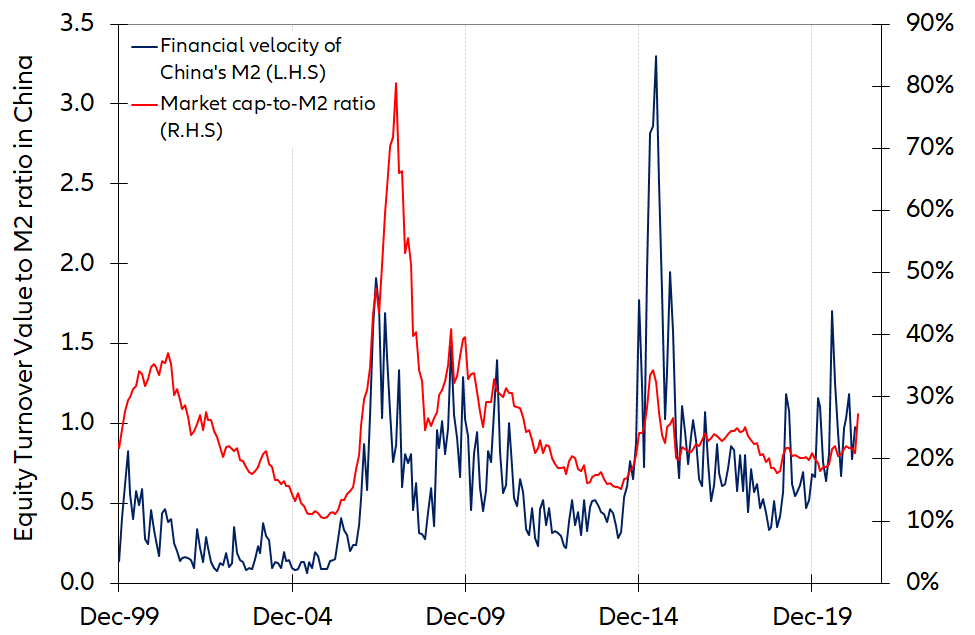
Our first key observation is that the financial velocity of money in China – defined as the ratio of equity turnover value-to-M2 – has been highly unstable1. From the trough to the peak of the last three market cycles, the financial velocity of money has been multiplied by almost 30 and 15, respectively, as shown in Table 1 and Figure 1.
Table 1 – Extreme values of M2 and its financial velocity through cycles